• Contour lines joins up points on a surface that are of the same height.
  • Field lines points towards the direction of the steepest descent.

As you may have noticed, whenever a field line and contour line crosses each other, they are perpendicular.

Prove it.

  • @Eheran
    2•27 days ago

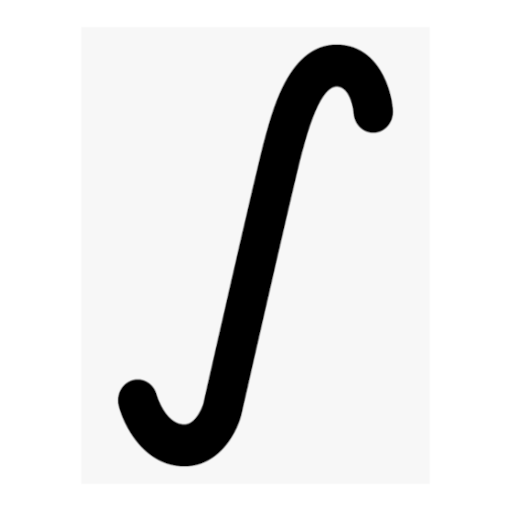
    Isn’t one calculated from the other? Surface gradient, so it is perpendicular by definition. Not sure if this is actually proven by measuring (or rather to what extent).

    • @[email protected]
      3•27 days ago

      The surface gradient is the part of a 3d (n dimensional) gradient of a scalar valued function (e.g. a flux vector) that remains within a 2d (n-1 dimensional) surface, i.e. without the contribution normal to the surface.

      The iso-lines, however, represent the regions where the function value remains constant. As the directional derivative, i.e. the scalar product of the gradient and the tangential vector of the curve must vanish, the gradient is orthogonal to those somewhat by definition. Yet, that the negative gradient is the direction of steepest descent, remains to be proven.